2.4 Exercises on differentials and comparative statics
- Find the differentials of the following.
- z = xy2 + x3.
- z = a1x12 + ... + anxn2 (where a1, ..., an are constants).
- z = A(α1x1−ρ + ... + αnxn−ρ)−1/ρ (where A, ρ, and α1, ..., αn are constants). [This is a constant elasticity of substitution function.]
- dz = (y2 + 3x2)dx + 2xy dy.
- dz = 2a1x1dx1 + ... + 2anxndxn.
- dz = A(α1x1−ρ + ... + αnxn−ρ)−1−1/ρ(α1x1−ρ−1dx1 + ... + αnxn−ρ−1dxn).
- Consider the system of equations
xu3 + v = y2 3uv − x = 4 - Take the differentials of both equations and solve for du and dv in terms of dx and dy.
- Find ∂u/∂x and ∂v/∂x using your result in part (a).
- Taking differentials of the equations we obtain
or, rearranging these equations,
u3dx + 3xu2du + dv = 2ydy 3vdu + 3udv − dx = 0 or3xu2du + dv = −u3dx + 2ydy 3vdu + 3udv = dx Inverting the matrix on the left, we obtain du = −((3u4 + 1)/D)dx + (6yu/D)dy and dv = ((3xu2 + 3u3v)/D)dx − (6yv/D)dy, where D = 9xu3 − 3v.
3xu2 1 
3v 3u 
du 
dv = 
−u3dx + 2ydy 
dx . - ∂u/∂x = −(3u4 + 1)/D and ∂v/∂x = (3xu2 + 3u3v)/D.
- The equilibrium values of the variables x, y, and λ are determined by the following set of three equations:
where p, q, and I are parameters and U is a twice differentiable function. Find ∂x/∂p. [Use Cramer's rule to solve the system of equations you obtain.]
U'1(x, y) = λp U'2(x, y) = λq px + qy = I Taking differentials we obtainor, setting dq = dI = 0,U"11(x,y)dx + U"12(x,y)dy = pdλ + λdp U"21(x,y)dx + U"22(x,y)dy = qdλ + λdq xdp + pdx + ydq + qdy = dI Using Cramer's rule we find that
U"11 U"12 −p 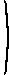
U"21 U"22 −q p q 0 
dx 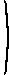
dy dλ = 
λdp 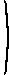
0 −xdp . dx = (λq2 + qxU"12 − pxU"22)dp/Δ,where Δ is the determinant of the matrix on the left-hand side of the equation, or∂x ∂p = λq2 + x(qU"12 − pU"22) Δ . - The equilibrium values of the variables Y, C, and I are given by the solution of the three equations
where T, G, and r are parameters and f and h are differentiable functions. How does Y change when T and G increase by equal amounts?
Y = C + I + G C = f(Y, T, r) I = h(Y, r) Taking differentials we obtainordY = dC + dI + dG dC = f'1(Y,T,r)dY + f'2(Y,T,r)dT + f'3(Y,T,r)dr dI = h'1(Y,r)dY + h'2(Y,r)dr, dY(1−f'1(Y,T,r) − h'1(Y,r)) = f'2(Y,T,r)dT + (f'3(Y,T,r) + h'2(Y,r))dr + dG,orThus if dT = dG and dr = 0 we havedY = f'2(Y,T,r)dT + (f'3(Y,T,r) + h'2(Y,r))dr + dG 1−f'1(Y,T,r) − h'1(Y,r) (Given that the question asks only about changes in T and G, we could ignore the change dr in r from the beginning.)dY = (1 + f'2(Y,T,r))dT 1−f'1(Y,T,r) − h'1(Y,r) - An industry consists of two firms. The optimal output of firm 1 depends on the output q2 of firm 2 and a parameter α of firm 1's cost function: q1 = f(q2,α), where f'1(q2,α) < 0 and
f'2(q2,α) > 0 for all q2 and all α. The optimal output of firm 2 depends on the output q1 of firm 1: q2 = g(q1), where g'(q1) < 0. The equilibrium values of
q1 and q2 are thus determined as the solution of the simultaneous equations
Is this information sufficient to determine whether an increase in α increases or decreases the equilibrium value of q1?
q1 = f(q2, α) q2 = g(q1). We haveso thatdq1 = f'1(q2,α)dq2 + f'2(q2,α)dα dq2 = g'(q1)dq1 ∂q1/∂α = f'2(q2,α) / [1−f'1(q2,α)g'(q1)].Since we don't know if f'1g' is less than or greater than 1, we don't know if this is positive or negative. - The variables x and y are determined by the following pair of equations:
where f, g, and h are given differentiable functions, β is a constant, and A is a parameter. By taking differentials, find ∂x/∂A and ∂y/∂A.
f(x) = g(y) Ay + h(x) = β We haveso that, solving these two equations for dx and dy we deduce thatf'(x)dx = g'(y)dy Ady + ydA + h'(x)dx = 0 ∂x/∂A = −g'(y)y/[Af'(x) + g'(y)h'(x)]and∂y/∂A = −f'(x)y/[Af'(x) + g'(y)h'(x)]. - The optimal advertising expenditure of politician 1 depends on the spending s2 of politician 2 and a parameter α: s1 = f(s2, α), where 0 < f'1(s2, α) < 1 and
f'2(s2, α) < 0 for all s2 and all α. The optimal expenditure of politician 2 depends on the spending s1 of politician 1 and a parameter β: s2 = g(s1, β), where 0 <
g'1(s1, β) < 1 and g'2(s1, β) < 0. The equilibrium values of s1 and s2 are given by the solution of the simultaneous equations
Does an increase in α (holding β constant) necessarily increase or necessarily decrease the equilibrium value of s1?
s1 = f(s2, α) s2 = g(s1, β). We haveso thatds1 = f'1(s2, α)ds2 + f'2(s2, α)dα ds2 = g'1(s1, β)ds1 + g'2(s1, β)dβ ∂s1/∂α = f'2(s2, α)/[1 − f'1(s2, α)g'1(s1, β)].Since 0 < f'1 < 1 and 0 < g'1 < 1 and f'2 < 0, an increase in α reduces the equilibrium value of s1. - The equilibrium outputs q1 and q2 of two firms satisfy
where b is a differentiable function that is decreasing in each of its arguments and satisfies b'1(q, c) > −1 for all q and c, and c1 and c2 are parameters.
q1 = b(q2, c1) q2 = b(q1, c2), - Find the differentials of the pair of equations.
- Find the effect on the values of q1 and q2 of equal increases in c1 and c2 starting from a situation in which c1 = c2 and an equilibrium in which q1 = q2.
- The differentials of the pair of equations are
dq1 = b'1(q2, c1)dq2 + b'2(q2, c1)dc1 dq2 = b'1(q1, c2)dq1 + b'2(q1, c2)dc2. - We may write these equations as
so that

1 −b'1(q2, c1) 
−b'1(q1, c2) 1 
dq1 
dq2 = 
b'2(q2, c1)dc1 
b'2(q1, c2)dc2 , where Δ = 1 − b'1(q2, c1)b'1(q1, c2). Hence
dq1 
dq2 = (1/Δ) 
1 b'1(q2, c1) 
b'1(q1, c2) 1 
b'2(q2, c1)dc1 
b'2(q1, c2)dc2 , dq1 = (1/Δ)[b'2(q2, c1)dc1 + b'1(q2, c1)b'2(q1, c2)dc2] dq2 = (1/Δ)[b'1(q1, c2)b'2(q2, c1)dc1 + b'2(q1, c2)dc2]. To find the effect on q1 and q2 of small and equal increases in c1 and c2, set dc1 = dc2 = dc. Given q1 = q2 = q and c1 = c2 = c we have b'2(q1, c2) = b'2(q2, c1), so that
so that dq1 = dq2 < 0. That is, both outputs decrease, and do so by the same amount.dq1 = (1/Δ)b'2(q, c)[1 + b'1(q, c)]dc dq2 = (1/Δ)b'2(q, c)[b'1(q, c) + 1]dc,
- Find the differentials of the pair of equations.
- The equilibrium values of the variables Y and r are given by the solution of the two equations
where a > 0 and M are parameters, I is an increasing differentiable function, and S and L are decreasing differentiable functions. How do Y and r change when M increases (holding a constant)?
I(r) = S(Y) aY + L(r) = M Taking differentials we obtainorI'(r)dr = S'(Y)dY adY + L'(r)dr = dM Solving for dr we get
I'(r) −S'(Y) 
L'(r) a 
dr 
dY = 
0 
dM . ∂r/∂M = S'(Y)/[aI'(r) + L'(r)S'(Y)] < 0.Similarly, solving for dY we get∂Y/∂M = I'(r)/[aI'(r) + L'(r)S'(Y)] > 0. - Consider a market containing two goods. Denote the prices of these goods by p and q. Suppose that the demand for each good depends on p, q, and the amount of advertising expenditure a on good 1, and that the supply of each good depends only on the price of that good. Denoting the demand functions by x and y and the supply functions by
s and t, for any given value of a a market equilibrium is a pair (p, q) or prices such that
x(p, q, a) = s(p) y(p, q, a) = t(q). How does the equilibrium price p of good 1 change as a changes?
Assume that x'p < 0, x'q > 0, x'a > 0, s' > 0, y'q < 0, y'p > 0, y'a < 0, and t' > 0. (What are the economic interpretations of these assumptions?) Assume also that (x'p − s')(y'q − t') − x'qy'p > 0 for all (p, q, a). Does the equilibrium price of good 1 necessarily increase if a increases?
Take differentials of the system of equations (noting that p and q are variables, and a is a parameter):x'p(p, q, a)dp + x'q(p, q, a)dq + x'a(p, q, a)da = s'(p)dp y'p(p, q, a)dp + y'q(p, q, a)dq + y'a(p, q, a)da = t'(q)dq. Now solve for dp and dq. Writing the system in matrix form (omitting the arguments of the functions), we have
so that
x'p − s' x'q 
y'p y'q − t' 
dp 
dq = 
−x'ada 
−y'ada , where Δ = (x'p − s')(y'q − t') − x'qy'p. Hence
dp 
dq = (1/Δ) 
y'q − t' −x'q 
−y'p x'p − s' 
−x'ada 
−y'ada , dp = (1/Δ)[−(y'q − t')x'a + x'qy'a]da.Under the stated assumptions, Δ > 0, but the coefficient of da is not necessarily positive—if x'q and/or y'a are large enough then it could be negative. Thus the price of good 1 may decrease when a increases: if the (positive) effect of an increase in a on the demand for good 1 is small while the (negative) effect on the demand for good 2 is large, then the equilibrium price of good 1 may fall when a increases.
If, however, x'qy'a is small then the equilibrium price of good 1 definitely increases.