Mathematical methods for economic theory

Martin J. Osborne
Previous section Previous page Contents Text Exercises Search Next page Next section

1.4 Exercises on intervals and functions

  1. At what points is the function
    f(x) = 
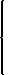
    left brace x2 − 2 if x ≤ 0
    −x2 if 0 < x < 1
    x2 − 2 if x ≥ 1
    continuous?

    Solution

    The function is continuous at all points except 0. We have f(0) = −2, but for x slightly greater than 0, f(x) is close to 0.

  2. Use the Intermediate Value Theorem to show that the equation x7 − 5x5 + x3 − 1 = 0 has a solution between −1 and 1.

    Solution

    Denoting the function by f, we have f(−1) = 2 > 0 and f(1) = −4 < 0. Thus by the Intermediate Value Theorem there exists x between −1 and 1 such that f(x) = 0.